John Golden's tweet made me think about pattern blocks and holes again:
My best shot at 8! pic.twitter.com/VipMdexBfB— Math Bad Hombre (@mathhombre) October 30, 2016
There's a nice kind of arithmetic with pattern blocks. For instance, these two houses have the same shape and size:
 |
| (Picture created on mathtoybox) |
Which tells us that the area of the square is equal to the area of the two rhombuses.
A dodecagon like this
can be made a lot of different ways (try it!) but they will all have an area equivalent to six squares and twelve triangles.
Which brings me to holes. Using these two bits of knowledge, we can say what area this hole in the dodecagon must have.
We've got the twelve triangles. We've got the equivalent of one square (in the form of the two thin rhombuses). So the spiky shape in the middle must be... five squares big. (In this case you can also see how five rhombuses and two squares would fill the space.)
So, thinking to enlarge the family, if the square is twice the thin rhombus, what would three times the thin rhombus look like? I used holes to find some. Here they are, coloured in non-pattern-block colours:
The pink S is just the shape of three thin rhombuses next to each other.
I like that purple S-shaped one!
The fuchsia one is the most obvious: a square and a thin rhombus joined.
[edit] Here's a claim. All those 1½-square holes are concave. I think there is no convex pattern-blocks-compatible convex shape (ie with unit-length sides, pattern block angles).
I'm thinking about alternative pattern blocks you see. I recently bought some deci-blocks:

They extend the triangle even further, in interesting ways;
— Mark Chubb (@MarkChubb3) October 31, 2016Christopher Danielson is thinking of other ways to create a beautiful new set of 21st century pattern blocks:
What I'm wondering here though, is, if there was a family for the thin rhombus, how would that look? Or should we just go for bigger members of the square family, with a domino, triomino, hexomino?This image from @Trianglemancsd would make a good #fractiontalke as well. The TL especially, but the others help @MarkChubb3 @FractionTalks pic.twitter.com/o9YH1w4349— Simon Gregg (@Simon_Gregg) October 31, 2016
And then I'm thinking the arithmetic of holes could be a good one for the older years/grades of primary/elementary or even beyond. These three dodecagons all have the same size hole. What size is it?
Then, getting a little harder, what size hole would this hole be?
And what about the two holes in this?
By the way, if you want to use my images yourself, go ahead.
- - - Update, March 2017 - - -
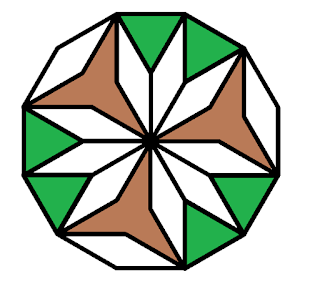
Looking at this tweet
made me think how "stars" could be good to play with:













Geometry is an area I still have a lot to learn. So I have been gratefully watching these fantastic discussions from the sidelines. Thanks for sharing Simon!
ReplyDelete