Liping Ma

I've been reading Liping Ma's 1999 Knowing and Teaching Elementary Mathematics, very relevant to the moment, for teachers in (or from!) England as much as teachers in the States. It compares the pedagogical subject knowledge of teachers in the US and in China. And finds that the Chinese teachers have got a lot more of it.

This is relevant to the English because there's a lot of similarity between English and American teachers, and the UK government is looking towards Shanghai for inspiration, and promoting a "mastery" curriculum. There is of course the PISA-envy we feel towards Shanghai and Singapore, and along with it plenty of doubts and caveats.

But, whatever your starting point as an elementary maths educator, there's a lot of good research and a lot to think about in this book. Here's two of my takeaways:

1. Depth of teacher knowledge

One of the things she does is look at how teachers would teach four scenarios. For instance subtraction. She looked at how teachers responded to this question:

Let’s spend some time thinking about one particular topic that you may work with when you teach: subtraction with regrouping. Look at these questions: 

 How would you approach these problems if you were teaching second grade? What would you say pupils would need to understand or be able to do before they could start learning subtraction with regrouping?

She found that lots of US teachers talked about "borrowing", while most Chinese teachers talked about regrouping, with lots talking about multiple ways of regrouping.

Have a look at Liping Ma talking about this:

She found that the Chinese teachers had a much better grasp of where this scenario fits within the whole curriculum. This subtraction questions for instance fitted in here:

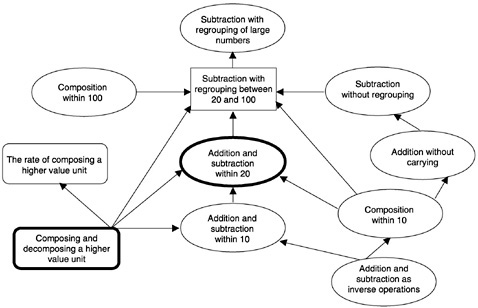
She gives a detailed description of what "profound understanding of fundamental mathematics" is. It's deep, broad and thorough.

"I define understanding a topic with depth as connecting it with more conceptually powerful ideas on the subject... understanding a topic with breadth, on the other hand , is to connect it with those of similar or less conceptual power." p121

I like this description of understanding and its importance. Though most of the book discusses the teaching of arithmetic, beyond the arithmetic is a deeper, algebraic understanding:

"There is a misunderstanding of arithmetic in this country. Many people think arithmetic equals computational skills, and that’s it. Arithmetic has a theoretical core and there is intellectual depth to it. That arithmetic can serve as a good foundation for students to learn algebra or other advanced math." (Interview in NY Times)

2. How teachers learn

My second takeaway is about how the Chinese teachers, who had a lot less school maths themselves, developed their profound understanding of fundamental mathematics.

They learnt mathematics:

1. studying the materials intensively;
2. from colleagues;
3. from students;
4. from doing mathematics.

The second jumped out. These teachers taught exclusively maths, and had plenty of time to talk about it with colleagues. I love these quotes on page 137:

"...I liked to listen to Xie and other teachers discussing how to solve a problem... I was so impressed that they could use seemingly very simple ideas to solve very complicated problems. It was from them that I started to see the beauty and power of mathematics." 

"In my teaching research group I am the oldest one... yet I learn a lot from my younger colleagues. They are usually more open-minded than I am..." 

"I think a teaching research group is always helpful because you need to be stimulated by someone else when trying to have a better understanding of something."

In my career the person who coordinates a subject in a school rarely gets extra time to do it, and usually doesn't have the time or the opportunity to talk over their subject on a regular basis. They don't get to visit classrooms, they don't get to discuss lessons. I plan with my partner teacher in the other Year 4 class, and that's a great time to share ideas, but the pace is fast, we don't have a lot of time for reflection.

I loved seeing how Kristin and teachers at  Richard A. Shields Elementary School have given us lots of pointers about looking at our teaching together, including spending time looking at the mathematics and how it can be approached.

But this sort of great work is so rare. How do we move to a situation where teachers are talking to each other about lessons, reflecting on how to improve?

I was also struck that the Chinese teachers said they learnt from students too. So, the teacher is standing at the front, using a textbook, orchestrating the lesson - there seems still in their lessons to be a lot of space for students' ideas. Liping Ma presents a techer (p138) wh says, "Sometimes the way of solving a problem proposed by a student is one I've never thought about..." and gives the example of when he was teaching the are of this shape with algebra:

A student puts their hand up, and suggests drawing a rectangle round the shape like this:

The teacher "caught" the idea and ran with it in the lesson.

-§-

The book throws up a lot of questions about how we as teachers deepen our knowledge. I haven't got the answers, but I think they're definitely worth thinking about...