Taking some things with me from Early Years

I'm leaving the three, four and five year olds, and heading up to Grade 2 (which in UK is called Year 3).

There's so much I love about the way we work with the youngest children, and I'm hoping I'll take some of that with me.

Just two of the things for now, illustrated with two photos from the last week of the summer term.

Estelle on Sports Day with students

We join in

We don't want that 'I am the knowing, observing, and assessing adult' hanging over the students. We break that down by playing alongside the children. Plus, we have fun, and see what it's like to do the things they're doing, and we get ideas.

Rachel at the making table with two students

We are quiet

(Not always of course)

We can sometimes be larger than life, charismatic and inspiring, but lots of the time we are quiet. The students have the agency. We are with them, but not dominating the situation. The students know what they want to achieve. It helps to have us there, and there can be good conversations, but mostly it's the students concentrating on what they're doing.

These are two of the things I want to take with me.

Play as the foundation

I get to see the richness of play on a daily basis with the 3, 4 and 5 year olds I work with. They learn through this play, even though it often looks very different to many an adult's idea of learning. It's through this play that their understanding of themselves and the physical and social world around them develops. They also learn what their powers are, how they can arrange things, make things happen, create important moments with others.

I've been thinking about how this is the perfect course to set off on to develop the kinds of abilities, or "competencies" that they will need as adults.

Play is notoriously difficult to define, but I think Peter Gray's definition makes sense. Play is:

1. Freely chosen & directed by the players
2. Intrinsically motivated 
3. Structured by rules within the player’s mind
4. Always creative & usually imaginative
5. Conducted in an active, alert, relatively non-stressed frame of mind

He elaborates on this, to show the power each of these characteristics for children's development and learning (my emphasis, in bold):

1. Because it is freely chosen and directed by the players, play is a major force for children’s learning how to take initiative, direct their own behavior, negotiate with and get along with playmates, and solve their own problems.
2. Because it is intrinsically motivated, play is how children discover, pursue, and become skilled at what they love to do.
3. Because it is guided by mental rules, play is how children learn to plan, structure, and create the boundaries (rules) for activities that engage them.
4. Because it is always creative and often highly imaginative, play is how children exercise and build their capacities for creativity and imagination.
5. Finally, the mental state of play—active and alert but relatively non-stressed—has been shown in many studies to be the ideal state of mind for learning anything new or doing anything that requires creativity or the generation of new insights.

I also came across this 'Learning Compass' from the OECD:

"Developed as part of our Future of Education and Skills 2030 project, the Learning Compass puts forth a shared vision of what students should learn to be ready for tomorrow."

There's some other important elements around the compass, which I've taken off; I wanted to focus on the compass circle itself.

Looking at the dark cyan ring - the 'transformative competencies' -

• Taking responsibility
• Reconciling tensions and dilemmas
• Creating new value

- I'm struck by how similar these are to the Gray's elaborations of aspects of play.

Just to make it clear, let's put them side by side:

Play	Transformative Competencies
play is how children learn to plan, structure, and create the boundaries (rules) for activities that engage them	Taking responsibility
take initiative, direct their own behaviour, negotiate with and get along with playmates, and solve their own problems	Reconciling tensions and dilemmas
play is how children exercise and build their capacities for creativity and imagination.	Creating new value

Play is developing the kinds of competencies with which we might hope students finish their schooling, those with which they not only know about the world, but can make positive changes to it.

It makes me think that play could be right in the middle of that compass! Play develops skills, knowledge, values and attitudes.

I'm thinking about play for young children here, but I'd like to see playful learning continuing beyond that age to maintain the self-directed approaches children have learnt so much from.

I think it's always useful to think about what that learning is like in the specific case. Luckily, I've blogged about some of these concrete examples. Here's a couple of links to earlier posts:

Arranging things - "What I’m trying to get a grip on doesn’t seem to simply reduce to dispositions though. It is a more disorganised-seeming, less direct way of obtaining knowledge about what daring and playfulness can achieve, what can be done with freedom and within necessity, how the social and physical environment can be remixed. It centres around agency, and uses whatever is at hand to achieve its undefined aims. It achieves its goal of developing capable and skillful being and making in the physical and social world, but its means are more indirect than what comes to mind when we think of theory-building: curiosity -> question -> search -> answers."

Folding, cutting, sticking, drawing - "...it's really not necessary for me to be adding anything in to this process: there's so much happening already: theories being refined, interests pursued, skills honed, and much more."

Mathematics Lessons to Look Forward To!

Jim's book is out!

Jim Noble is my friend and colleague in secondary. His classroom just a few metres from our Early Years playground, no doubt he's had to shut his door many times because of the racket we're making! I see him out there too. 

'If the world were a hundred people...'

Human Loci: Creating a parabola

These are part of two of the lessons described in Jim's Mathematics Lessons to Look Forward To! The book details twenty lessons that Jim returns to, experiments with, hones and polishes. 

"Every time I revisit a topic for myself or in preparation for teaching or mostly during teaching, I always notice something I haven’t seen before and this is often pointed out by a student."

Jim is a great storyteller. He's often called on to be the one who puts important rites of passage in the life of the school into words: leaving speeches, introducing speakers and celebrations. He's always assured, natural, entertaining and considered in what he says. 

This book is the same. And Jim is letting us into the heart of his teaching here, there's a vulnerability, at times, a touch of self-doubt or self-mockery. In the process, he takes us back to what makes the lessons tick for him, why they became exciting and vital.

"Deep down I have convinced myself that the roots of ideas are an important part of them. I think the journey from first idea to activity is a really enjoyable, reflective part of the job."

His lessons are not always outdoors of course, but they are all out of the routine, they all stand out as being alive, practical where possible... experiences as well as lessons. And fun.

"It is fun. I have made no apology about this. I have found that I need this as much as students do. Something that adds variety to the global experience, something practical that gets students out of their seats and sometimes out of the classroom and something that makes us laugh a little is always welcome."

Reading a draft of the book, I was struck again by how we have so much in common in our outlook towards lessons. Is it that we've spent a lot of time together since 2004 when Jim came to the International School of Toulouse?

It's not just that we both photograph drain covers for their mathematical patterns, both enjoy seeing and making Islamic geometrical patterns, both value Seymore Pappert's seminal book Mindstorms...

Many - most! - of his twenty lessons do actually have their counterpart in the primary school.

We've both enjoyed 'Numbersearch' and these feature in the book:

If the white triangle is 1, what other numbers can you see?

Jim got pi involved:

If the white square is one...

Like Jim in secondary, I love to get my primary students coding the path of a robot using Scratch:

“If I need to turn 5 equal turns that make a total of 360 then I need to turn 72 degrees”, which generalises to “for an n sided regular polygon, the turning angle is 360/n”. The thrill of making this conclusion is the same thrill as solving a puzzle. The word ‘discovery’ is much maligned and probably inappropriate as it implies a kind of wandering around until you find something then pick it up. This is much more mathematical in nature. You have a problem that needs solving, you have knowledge of the scenario at the ready and you put bits of this knowledge together to deduce new knowledge. Now that is doing mathematics.

Or using dynamic geometry:

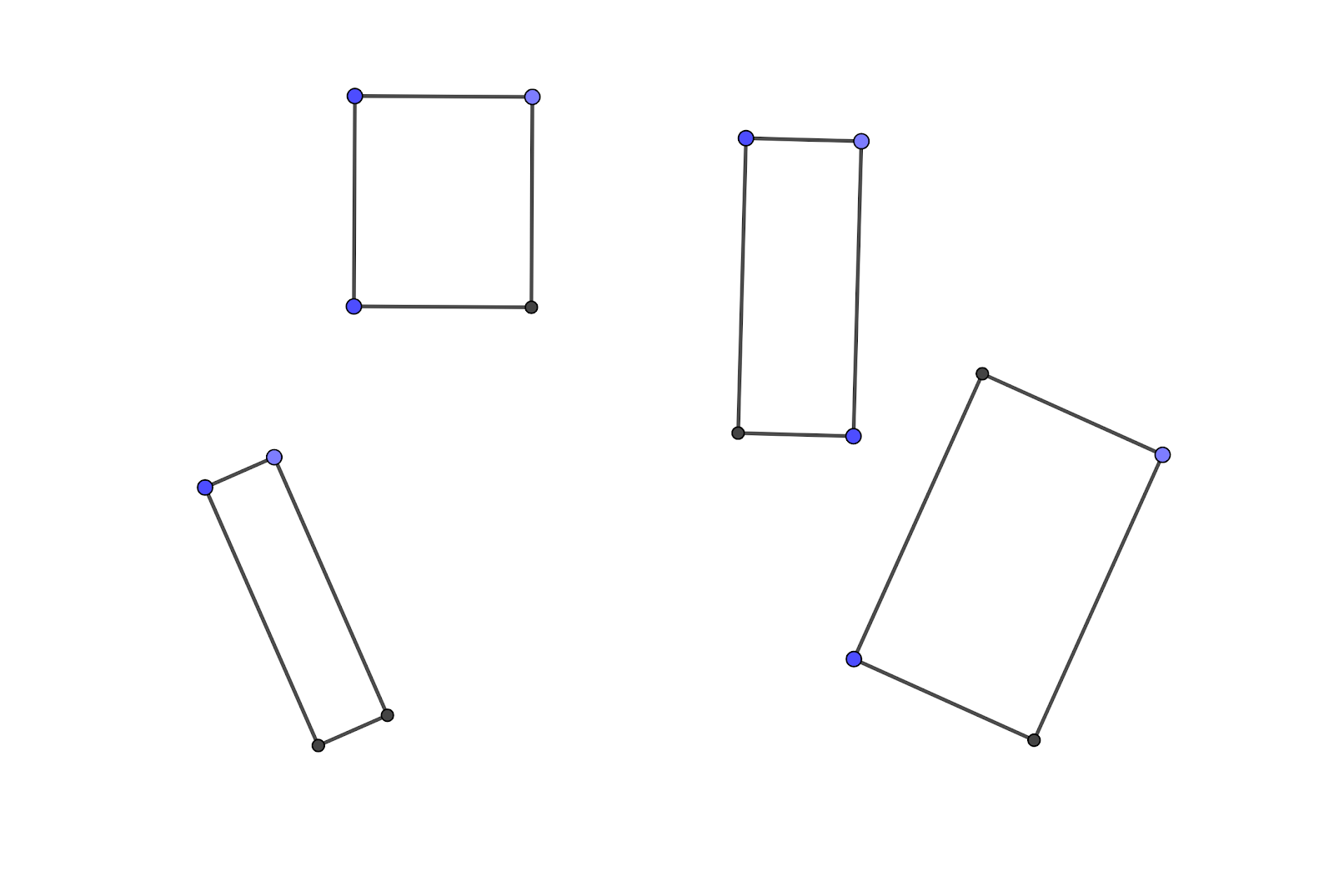
How would you construct this rectangle? The others? I really do recommend having a go here. It is really interesting to focus on the different ways it can be done and there are some surprising challenges hidden away in there.

In this activity, the constructed dynamic rectangle is, in a sense, every rectangle. Students get to explore the notion that each construction has a degree of freedom that is entirely defined by the elements that were used to construct it and the order in which they were used. It is a profound mathematical idea that goes beyond geometry into set theory and the anatomy of a variable. In many ways it is a much more natural way to see mathematics that a set of static images might be and really helps get our heads around the idea of generalisation. “Many things here can vary, but the following will always be true”

Jim asks the reader to have a go at parts the activities. Some of them I tried. I had a go for instance at constructing a rectangle four different ways using Geogebra. Each one has a different 'skeleton', the way it's constructed.

(I've come to the conclusion there's an infinite way of constructing a rectangle that can be stretched into any rectangle and these skeletons don't have to involve parallel sides, or perpendicular sides either.)

There is really no other book like this! Jim takes each lesson from his treasure chest of pedagogical subject knowledge and turns it every way in the light for us. Which different ways could the lesson go? How does it relate to key ideas in mathematics? How does it relate to our understanding of what it means to know? How does it engage students? What connections are there with other subjects?

Jim showing piles of rice to Helen and Mike

You can tell I'm recommending it!

Great teacher, great book!

The lesson chapters:

 1 - What’s in the box

 2 - Cones

 3 - If the world was a village of 100 people

 4 - Goodness Gracious Great Piles of Rice

 5 - How do I love thee, let me count the ways

 6 - Number Searches

 7 - Human Loci

 8 - Statistics telling stories

 9 - Match Point

 10 - Prime Pictures

 11 - Population Growth

 12 - Starting from scratch

 13 - Indestructible

 14 - Dancing Quadratics

 15 - Hot Wheels

 16 - Maxbox

 17 - Dancing Vectors

 18 - Pleasure at the Fairground

 19 - Impossible Diagrams

 20 - Cubism